What Is A Vertical Slope

Dissimilar words, same formula

Example One

The slope of a line going through the point (1, 2) and the indicate (iv, 3) is $$ \frac{1}{3}$$.

Remember: difference in the y values goes in the numerator of formula, and the departure in the x values goes in denominator of the formula.

Can either signal be $$( x_1 , y_1 ) $$ ?

There is only 1 way to know!

The work , next

point (4, 3) as $$ (x_1, y_1 )$$

$$ slope = \frac{y_{ii}-y_{1}}{x_{2}-x_{1}} = \frac{three-2}{4-1} = \frac{1}{3} $$

point (1, 2) as $$ (x_1, y_1 )$$

$$ slope = \frac{y_{two}-y_{1}}{x_{two}-x_{one}} = \frac{2-three}{1-iv} = \frac{-1}{-3} = \frac{i}{three} $$

Answer: It does not affair which point you put first. You lot can start with (iv, 3) or with (1, 2) and, either way, you end with the exact same number! $$ \frac{i}{iii} $$

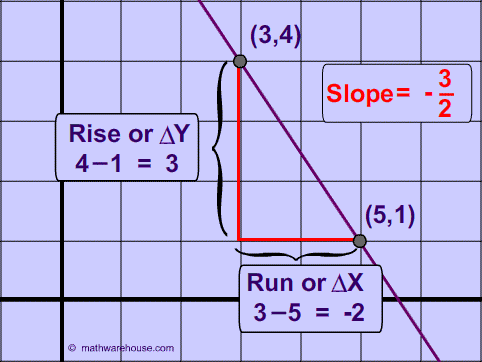
Example two of the Slope of A line

The slope of a line through the points (3, 4) and (5, 1) is $$- \frac{3}{two}$$ because every time that the line goes down by 3(the alter in y or the rise) the line moves to the right (the run) by 2.

Video Tutorial on the Slope of a Line

Slope of vertical and horizontal lines

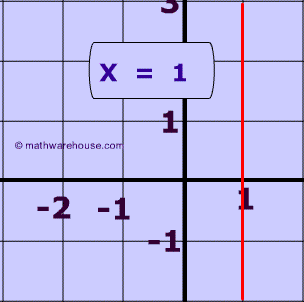
The slope of a vertical line is undefined

This is because whatsoever vertical line has a $$\Delta ten$$ or "run" of zero. Whenever zero is the denominator of the fraction in this case of the fraction representing the slope of a line, the fraction is undefined. The picture below shows a vertical line (x = 1).

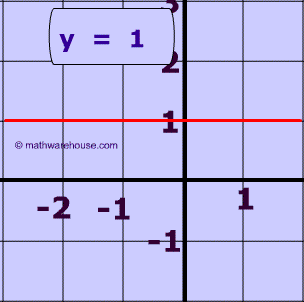
The gradient of a horizontal line is zero

This is considering whatever horizontal line has a $$\Delta y$$ or "rise" of cypher. Therefore, regardless of what the run is (provided its' not besides zero!), the fraction representing gradient has a null in its numerator. Therefore, the gradient must evaluate to zero. Below is a picture of a horizontal line -- you can run across that it does non take whatever 'rising' to information technology.

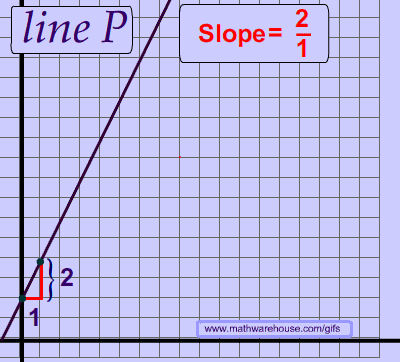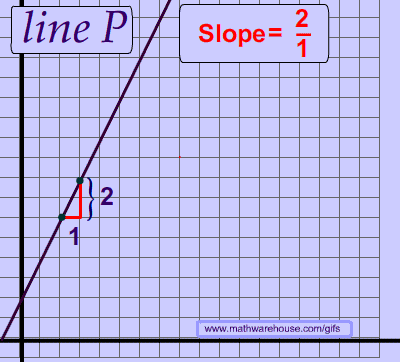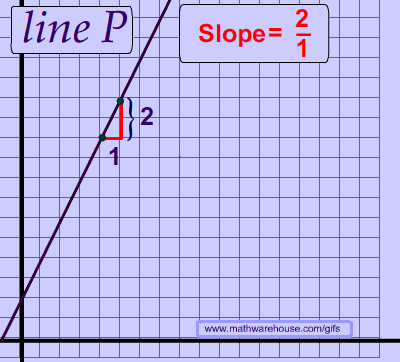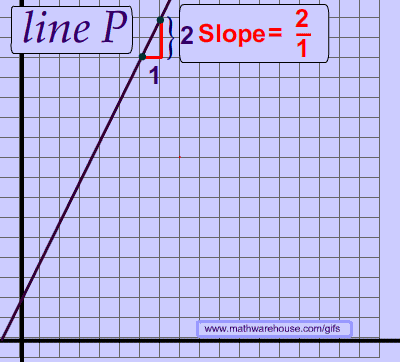
Do any two points on a line have the aforementioned slope?

Respond: Yes, and this is a primal point to remember about calculating slope.

Every line has a consistent gradient. In other words, the slope of a line never changes. This cardinal thought means that you can choose any ii points on a line.

Slope is consistent

Think about the idea of a direct line. If the gradient of a line inverse, then information technology would be a zigzag line and not a straight line, as you lot can come across in the picture above.

As yous can encounter below, the slope is the same no matter which 2 points you chose.

The Gradient of a Line Never Changes

Slope never changes animated gif

Practise Problems

Trouble 1

What is the gradient of a line that goes through the points (x,3) and (vii, 9)?

$ \frac{ascension}{run}= \frac{y_{2}-y_{one}}{x_{ii}-x_{one}} $

Using $$ \ruby{ (10,3)}$$ as $$x_1, y_1$$

$ \frac{9- \red 3}{seven- \blood-red{10}} \\ = \frac{6}{-iii} \\ = \boxed {-2 } $

Using $$ \red{ (7,9)} $$ every bit $$x_1, y_1$$

$ \frac{3- \reddish 9}{10- \red 7} \\ =\frac{-vi}{3} \\ = \boxed{-2 } $

Trouble ii

A line passes through (4, -2) and (4, three). What is its slope?

$ \frac{rise}{run}= \frac{y_{2}-y_{i}}{x_{two}-x_{1}} $

Using $$ \carmine{ ( 4,3 )}$$ equally $$x_1, y_1$$

$ = \frac{-2 - \red 3}{4- \carmine 4} \\ = \frac{-5}{ \color{cherry-red}{0}} \\ = \text{undefined} $

Using $$ \blood-red{ ( four, -two )}$$ equally $$x_1, y_1$$

$ = \frac{three- \red{-2}}{4- \crimson 4} \\ = \frac{5}{ \color{scarlet}{0}} \\ = \text{undefined} $

Whenever the run of a line is zero, the slope is undefined. This is because there is a nil in the denominator of the slope! Any the gradient of any vertical line is undefined .

Problem 3

A line passes through (2, 10) and (8, 7). What is its slope?

$ \frac{rising}{run}= \frac{y_{two}-y_{1}}{x_{2}-x_{one}} $

Using $$ \cerise{ ( 8, 7 )}$$ equally $$x_1, y_1$$

$ \frac{ten - \scarlet vii}{2 - \ruby 8} \\ = \frac{3}{-six} \\ = -\frac{1}{two} $

Using $$ \cherry{ ( 2,10 )}$$ as $$x_1, y_1$$

$ \frac{7 - \red {ten}}{8- \carmine ii} \\ = \frac{-3}{6} \\ = -\frac{one}{2} $

Problem 4

A line passes through (7, 3) and (8, v). What is its slope?

$ \frac{rise}{run}= \frac{y_{ii}-y_{1}}{x_{2}-x_{1}} $

Using $$ \cherry{ (7,iii )}$$ as $$x_1, y_1$$

$$ \frac{ v- \red 3}{8- \ruby 7} \\ = \frac{2}{1} \\ = 2 $$

Using $$ \carmine{ ( 8,five )}$$ as $$x_1, y_1$$

$$ \frac{ iii- \crimson 5}{seven- \reddish viii} \\= \frac{-2}{-1} \\ = 2 $$

Trouble 5

A line passes through (12, 11) and (9, 5) . What is its gradient?

$ \frac{rise}{run}= \frac{y_{2}-y_{1}}{x_{ii}-x_{1}} $

Using $$ \red{ ( five, 9)}$$ as $$x_1, y_1$$

$$ \frac{ eleven - \red v}{12- \carmine 9} \\ = \frac{6}{iii} \\ =2 $$

Using $$ \red{ (12, 11 )}$$ equally $$x_1, y_1$$

$$ \frac{ 5- \scarlet{ 11} }{9- \scarlet { 12}} \\ = \frac{-vi}{-3} \\ = 2 $$

Problem 6

What is the slope of a line that goes through (4, 2) and (iv, 5)?

$ \frac{rising}{run}= \frac{y_{2}-y_{ane}}{x_{2}-x_{i}} $

Using $$ \red{ ( 4,5 )}$$ as $$x_1, y_1$$

$$ \frac{ 2 - \reddish 5}{iv- \ruby 4} \\ = \frac{ -3}{\color{cherry}{0}} \\ = undefined $$

Using $$ \cherry-red{ ( 4,2 )}$$ as $$x_1, y_1$$

$$ \frac{ v - \red 2}{4- \red iv} \\ = \frac{ 3}{\colour{crimson}{0}} \\ = undefined $$

WARNING! Can you catch the error in the following problem Jennifer was trying to observe the slope that goes through the points $$(\colour{blue}{1},\color{carmine}{3})$$ and $$ (\color{blue}{ii}, \colour{red}{6})$$ . She was having a chip of trouble applying the gradient formula, tried to summate slope three times, and she came up with 3 unlike answers. Can you determine the correct reply?

Claiming Problem

Find the gradient of A line Given Two Points.

Endeavor #1

$ slope= \frac{rise}{run} \\= \frac{\colour{cerise}{y_{ii}-y_{1}}}{\color{blueish}{x_{2}-x_{1}}} \\= \frac{half-dozen-3}{one-2} \\= \frac{3}{-1} =\boxed{-3} $

Endeavour #2

$$ slope= \frac{ascent}{run} \\= \frac{\colour{red}{y_{two}-y_{i}}}{\color{bluish}{x_{2}-x_{1}}} \\= \frac{6-3}{2-1} \\= \frac{3}{1} \\ = \boxed{3} $$

Attempt #3

$$ slope = \frac{rise}{run} \\= \frac{\color{ruby}{y_{2}-y_{i}}}{\color{blueish}{x_{2}-x_{i}}} \\ =\frac{ii-1}{6-3} \\ =\boxed{ \frac{one}{iii}} $$

The right reply is try #2.

In attempt #1, she did not consistently utilise the points. What she did, in attempt one, was :

$$ \frac{\color{cherry-red}{y{\boxed{_2}}-y_{1}}}{\color{blue}{ten\boxed{_{1}}-x_{2}}} $$

The problem with effort #3 was reversing the rise and run. She put the ten values in the numerator( top) and the y values in the denominator which, of course, is the opposite!

$$ \cancel {\frac{\color{blueish}{x_{2}-x_{1}}}{\colour{blood-red}{y_{two}-y_{1}}}} $$

Gradient Practice Trouble Generator

Yous can practice solving this sort of trouble as much as you would like with the slope problem generator below.

It will randomly generate numbers and inquire for the slope of the line through those two points. You can chose how big the numbers volition be by adjusting the difficulty level.